Discrete Math Proof Practice Problems

09 May, 2021

D A C C B. These problems are collections of home works quizzes and exams over the past few years.

Pin On Mathematik

Most of the questions on the final will be small variations on problems you have already seen on previous exams or problem sets.

Discrete math proof practice problems. A A B A B C b A B C A B c A B C A C. I am on the first one still I currently have. Your fluency with standard proof techniques including proof-by-contradiction.

M n 9r2N. Discrete Mathematics. 1 1 13 4 135 9 1357 16 13579 25.

P q r p q p r T T T F T T F T T F T F T F F T F T T F F T F F F F T T F F F T 2. In this video we tackle a divisbility proof and then prove that all integers are the difference of two squaresLIKE AND SHARE THE VIDEO IF IT HELPEDVisit ou. If x2R then x4 x 4.

Most of the problems are from Discrete Mathematics with ap-plications by H. Certainly we cannot draw that conclusion from just the few above examples. S c Hypothesis 2.

Practice Use a direct proof a contrapositive proof or a proof by contradiction to prove each of the following propositions. E B A C A B C A. Prove that log 2 3 is irrational.

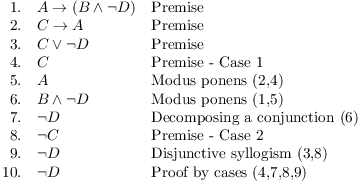
Discrete Mathematics First Order Logic Rules of Inference 1534 Formal Proof Using Inference Rules 1. If a b 19 then a 10 or b 10. The converse is If I drive to work today then it will rain The contrapositive is If I do not drive to work today then it will not rain.

Discrete Mathematics Inductive proofs Saad Mneimneh 1 A weird proof Contemplate the following. Proposition Suppose ab 2Z. Is l Dillig CS311H.

If it is raining then 11 Vacuous Proof. Input two bits xy and output two bits representing xy 11 00 10 01 0 0 00 01 11. Doing well on these questions will make a strong case for earning at least a B in the class.

Discrete math practice problems provides a comprehensive and comprehensive pathway for students to see progress after the end of each module. Notice that you are expected to understand inductionrecursion divisibility and modular arithmetic sets and functions in the abstract and basic principles of counting. But let us attempt to prove it.

Assume that the statement is false. If we know 푝푝 is false then 푝푝 푞푞 is true as well. But theres a special relationship between proofs and discrete math.

Proposition Suppose n is a composite integer. For the two statements below decide whether they are true or false. 8m2Nm.

Is l Dillig CS311H. Thus 3n 2 is even. X A B so x A or x B.

That is a 2k 1 and b 2m 1 for some integers k and m. These applets accompany the textbook Discrete Mathematics. Input three bits xyz and output one bit which is the majority of the three input bits.

Mathematical Reasoning and Proof with Puzzles Patterns and Games by Doug Ensley and Winston Crawley published by John Wiley and Sons. Students must understand mathematical reasoning in order to read comprehend and construct. The latter implies that n 2k for some integer k so that 3n 2 32k 2 23k 1.

It looks like the sum of the ﬁrst n odd integers is n2. With a team of extremely dedicated and quality lecturers discrete math practice problems will not only be a place to share knowledge but also to help students get inspired to explore and discover many creative ideas from themselves. If I am both rich and poor then 2 2 5.

Here are some problems chosen from my old Discrete Math exams which Id consider good practice but probably too easy for a Combinatorics exam. Therefore ab is odd. If we know 푞푞 is true then 푝푝 푞푞 is true as well.

If a b mod n and c d mod n then a c b d mod n. Then we have 3n 2 is odd and n is even. The development of some material on this site was funded by.

Ab 2k 12m 1 4km 2k 2m 1 22km k m 1. Input two bits xy and output two bits representing the absolute value of xy 3. In the Goals of a Discrete Mathematics Course section in the preface to his textbook Rosen puts Mathematical Reasoning first in the list.

Assume that a or b is even - say it is a the case where b is even will be identical. If ab is an even number then a or b is even. Ive deleted problems about.

Proposition Suppose abcd 2Z and n 2N. Let A B and C be sets. Discrete Math I Answers to Practice Problems for Exam I 1.

Discrete Mathematics First Order Logic Rules of Inference 1634 Another Example. If you want to read up on more types of proofs or Discrete Math topics in general a great book to easily learn and practice these topics is Practice Problems in Discrete Mathematics by Bojana Obrenic and Discrete Math Workbook. Interactive Exercises by James R.

Suppose a and b are odd. Proof by Contradiction Example 1 Show that if 3n 2 is an odd integer then n is odd. Discrete math practice problems provides a comprehensive and comprehensive pathway for students to see progress after the end of each module.

We hope that these notes will prepare a student to better understand basic mathematics necessary of. Proof-writing skills are important for all college-level math.

Conditional Statements Logic Foldable And Cheat Sheet Discrete Mathematics Rhetorical Analysis Essay Math Practice Worksheets

Discrete Mathematics Direct Proofs Examples Youtube

Proof By Contradiction Discrete Mathematics Youtube

Proof By Cases Explained W 5 Logic Examples

No Title Logic Discrete Mathematics Philosophy Quotes

Proof By Cases

Pin On Programming Languages

Pin On Mathematics

Logic Inb Pages High School Math Lesson Plans Geometry Interactive Notebook Math Interactive

Direct Proofs Discrete Mathematics Youtube

Chapter 1 The Foundations Logic And Proofs Discrete Mathematics And Its Applications Cse 211 Department Of Computer Sc Discrete Mathematics Mathematics Logic